PARABOLA’S PARAMETERS
EFFECTS
By Dario Gonzalez
Everyone who is familiar with functions knows that the general equation for any quadratic functions is:
The idea now is to
explore the effects of each parameter 
and 
in the graph a quadratic function. First, we are going to review some basic
transformation when the quadratic function’s equation is written as follow:
Expression (1)
It is precise to
emphasize that every quadratic function can be written as the Expression (1) by
following the completion of a square
method. That is, we can go from the
general expression to Expression (1) by doing as follow:
Here, we can consider:
and 
Then, we obtain the
Expression (1) again. This is a very
crucial fact since the Expression (1) can represent all the possible
transformation of the graph a quadratic function.
Given that most of you
are probably familiar with this fact, we will do a brief summary of the effects
produced by parameters 
and 
in the graph of the quadratic function.
EFFECT OF PARAMETER 
:
If we consider the quadratic function
where the parameters 
and 
,
and we make parameter 
varies; by considering the function 
as a reference, we will observe the effects of
parameter a in the graph of 
in the animation 1 below:
|
|
|
Animation 1 |
From figure 1 we can conclude the
effects of parameter if we consider as a reference the function in the following table below:
|
Sign of |
Positive |
Parabola is
opened upward |
|
Negative |
Parabola is
opened downward |
|
|
Value of |
|
Parabola
becomes broader |
|
|
Parabola
becomes steep |
EFFECTS
OF PARAMETER 
:
Now we are going to consider the quadratic function equation where
and . That is, taking as a reference the function , we can see the
graphic effects of parameter h in 
by observing the following animation 2:
|
|
|
Animation 2 |
Then, we can summary the effects of
parameter 
in the table below:
|
Sign of |
Positive |
Right move |
|
Negative |
Left move |
EFFECTS
OF PARAMETER 
:
Finally, if we consider a quadratic
function whose parameters and and the function as a reference, we visualize the graphic
effect of parameter k for the function 
in animation 3 below:
|
|
|
Animation 3 |
Thus, we can summary the effects of
parameter in the table below:
|
Sign of |
Positive |
Move up |
|
Negative |
Move down |
In general, we can conclude that for
each function f(x) it is possible to make the following transformations:
|
Parameter |
Relationship |
Characteristic |
Graphic effect |
|
|
|
--------- |
Reflection
around of X axe of f(x) graph |
|
|
|
|
The images of
f(x) increase in a |
|
|
|
The images of
f(x) decrease in a |
|
|
|
|
Positive |
The graph of
f(x) moves right |
|
|
Negative |
The graph of
f(x) moves left |
|
|
|
|
Positive |
The graph of f(x)
moves up |
|
|
Negative |
The graph of
f(x) moves down |
Even though this explanation is enough
to solve problems from 1 through 6, I would like to offer a different
approach. This approach considers all
what we review before and the graphic sum.
GRAPHIC
SUM:
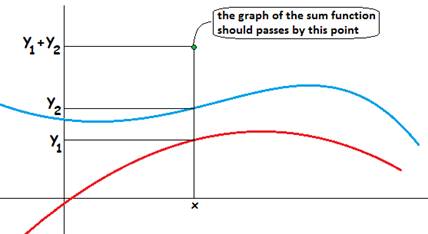
Graphic sum consists of obtaining the
graph of the sum function given the graphs of two functions. In other words, we consider the image of each
function in a fixed x, and we sum these two images obtaining one image if the
sum function for that fixed x. This
concept is shown in figure 1 below:
|
|
|
Figure 1 |
We must consider some important
observations:
Observation
1:
Let h(x) = f(x) + g(x), then h(x) = f(x) if g(x) = 0.
Observation
2:
Let h(x) = f(x) + g(x), then h(x) is going to behave like f(x) if |g(x)| is really small.
ANOTHER
APPROACH:
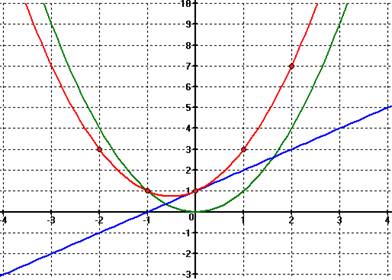
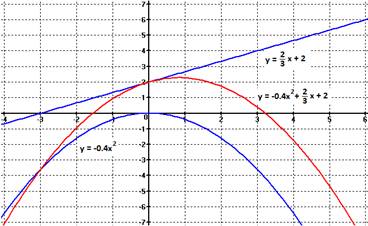
Consider the functions and 
whose graphs are shown in the figure 2 below:
|
|
|
Figure 2 |
We should highlight some important
issues in the sum of these two functions.
Issue
1: The sum function is going to intersect the linear function at x = 0, according to observation 1. Also, the sum function will behave similar to
linear function close to x = 0 because, according to observation2, the images
of the parabola are really small. It is important to note that the intersection
between the linear function and the sum function is tangential in x = 0. Indeed, if we analyze the values of the
functions f(x) and g(x) around x = 0,
we can see the values of the sum function will be slightly larger than the
linear function’s values. The only
exception occurs at x = 0 where both functions, the linear function and the sum
function, are equal.
Issue
2: The images of are going to be much larger than the images of
when the value of x goes to infinity; that is,
when 
the sum function will behave like a parabola
because the values of the linear function will be small in comparison to the
values of the parabola (observation 2).
Issue
3: Given the algebraic sum between and does not modify the coefficient of 
, we can conclude
that the sum function, which is going to be another parabola, has the same breadth of , according to
analysis of the parameters’ effects done above.
So, given the issues above mentioned,
the graph of the sum function is going to be a parabola like , but this sum
function will be tangent to the linear function in x = 0.
The graph of the sum function is shown below:
|
|
|
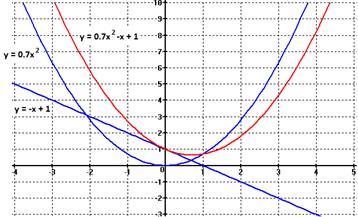
Figure 3 |
I plotted the graph of the sum
function with the graphs of the others function because I would like you to
appreciate how the sum graph has exactly the same shape that the parabola f(x),
but it is positioned on the linear
function .
Given that our above reasoning and
according to observation 2 and issue 1, we can conclude that even though the
green parabola could have been any parabola of the family , the sum
function would have had the same behavior.
In other words, it does not matter what is the value of parameter , if we do the
sum between and , we would have
obtained the same result; that is, the sum function will be a parabola like , but it would
have been tangentially positioned on
the linear function .
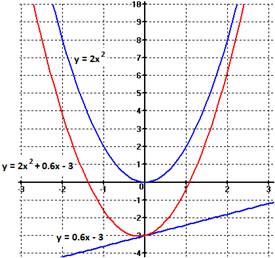
However, we can go further with this
conclusion. Observe the graphs shown in
figure 4. The blue graphs are the addend
functions, and the red graph is the sum function.
|
Figure 4 (a) |
|
|
Figure 4 (b) |
|
|
Figure 4 (c) |
|
After observing these graphs, we can
realize that the behavior of the sum function between a parabola and a linear function 
could be generalized in the following
statement:
Statement
1: Given a parabola and a linear function , the graph of
the function h(x) = f(x) + g(x) is a parabola like , but its graph
will be tangentially positioned on
the linear function in x = 0.
In other words, 
is a parabola tangent to at x = 0.
That statement establishes a crucial
base for our analysis about of the effects of the parameters and in the graph of the quadratic function . That is, we could consider the quadratic
function h(x) as a sum of two functions and , and from this
assumption, we can draw a interesting analysis of the parameters and .
EFFECTS
OF PARAMETERS 
AND 
:
The analysis developed above will
allow us to examine the graphic effects of the parameters and in the quadratic function .
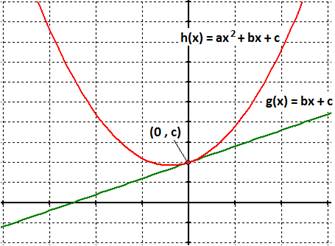
1) Parameter : First of all, by
appreciating figure 4 (a), (b) and (c), we reason that the parameter has two graphic representations: on the one
hand, represents the y-intersection for . On the other hand, determines the tangential intercept point
between the linear function 
and the quadratic function .
It is effortless to reach the anterior
conclusion if we consider the function as sum of and . We know the parameter in the linear function g(x) represents the
y-intercept, which occurs at x = 0 because 
. Also, we know that 
, then 
. Thus, also represents the y-intersection for h(x).
Moreover, we show easily that the
interception between h(x) and g(x) is tangential and occurs at
. To find the interceptions between h(x) and
g(x) we must solve the equation:
So, there exist exactly one
intersection between h(x) and g(x) that occurs at x = 0, and, given that 
, this intersection
appears at .
|
|
|
Figure 5 |
You can observe the following
animation 4 to visualize graphically the effects of parameter c.
|
|
|
Animation 4 |
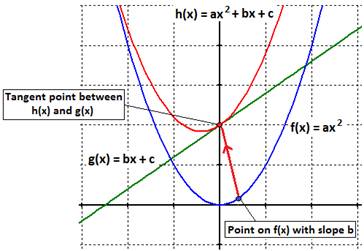
2) Parameter 
: Recall statement
1 and issue 3, we are capable to examine the graphic effect of parameter 
. The graph of a quadratic function is just a translation of the graph of the parabola . How we already know, h(x) is tangent to g(x)
at x = 0, then must have slope at x = 0.
Thus, h(x) is the translation of in a way that the point with slope on f(x) must intercept g(x)
at .
|
|
|
Figure 6 |
If we make parameter varies and fix parameters and , the slope of
g(x) is going to change, and the graph of g(x) is going to act as a seesaw.
So, given that h(x) is tangent to g(x) at x = 0, we can visualize the
graph of h(x) seems to glide along the linear function g(x) as follow
in animation 5 below:
|
|
|
Animation 5 |
This effect of the parameter rest upon the fact that the slope of only depend of this parameter when . In other words, the instantaneous change rate
of h(x) is when . Hence,
Thus,
Therefore, the parabola h(x) moves along the linear function g(x)
because the parabola h(x) has to keep tangentially touching g(x) at .
3) Parameter : We already know that the graph of a parabola represents a translation of the graph of a
parabola such that the point on the graph of f(x) that
has slope is translated to be a tangential intersection
with the liner function at x = 0.
Thus, we can analyze the effects of the parameter by observing the effects in , which we
already did, and considering the relationship above explained between h(x) and
g(x).
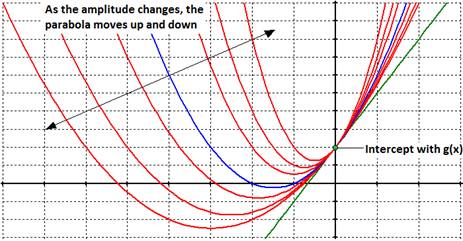
The parameter modifies the breadth and the orientation of like it was shown in figure 1. Consider to be positive and vary whereas we fix the
other parameters 
and 
. As we know, the breadth of the parabola f(x)
will change, and given that the statement 1 still holds, the effects of
parameter on the graph of can be described as an up-down movement around
the liner function g(x). That is, when 
h(x) becomes broader and moves down around the
linear function g(x). If 
h(x) becomes steep and moves up around he
linear function g(x). This is shown in
the animation 6 below:
|
|
|
Animation 6 |
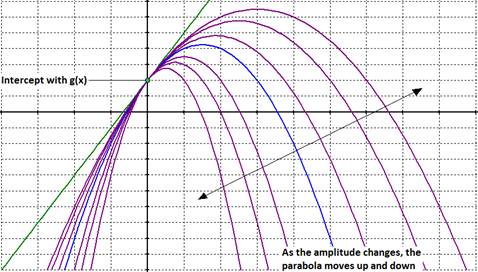
Here, in figure 7 below, there is a sort of a summary picture that shows a comparison among parabolas that have
different values for parameter a. The graph reference is the graph of , which is
presented by the blue parabola.
|
|
|
Figure 7 |
Now if we consider negative, and we have 
then the parabola h(x) becomes broader and
move up around the liner function g(x).
If 
then the parabola h(x) becomes steep and moves
down around the liner function g(x).
This is shown is animation 7 below:
|
|
|
Animation 7 |
Here, in figure 8 below, there is a sort of a summary picture that shows a comparison among parabolas that have
different values for parameter a (now for negative values). The graph reference is the graph of 
, which is
presented by the blue parabola.
|
|
|
Figure 8 |
Of course,
if parameter a = 0 the parabola becomes into a linear function
and, for this case, the “linear” parabola coincides with the linear function g(x)
= bx + c.
FINAL COMMENTS
I would like to highlight some final comments according to what we
have been analyzing here.
Comment 1: The functions and have the same value for their first derivative
at x = 0.
Comment 2: The function will have a root at x = 0 if and only if c =
0.
Comment 3: The vertex of
the function will be the intersection point with the linear
function if and only if b = 0.
